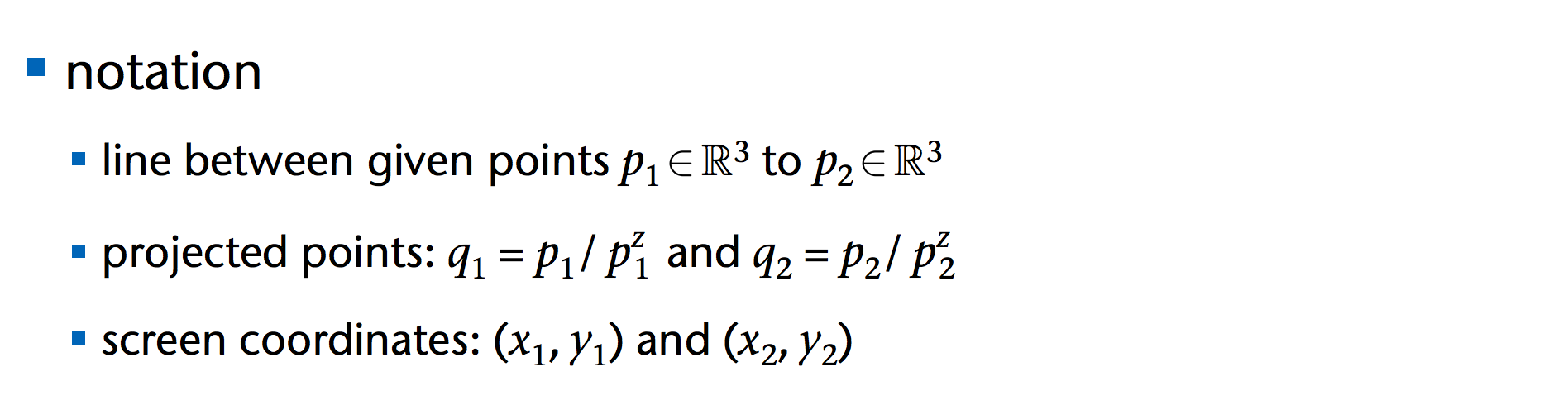Projected points and screen coordinates

Question

I'm trying to understand part of the contents of a slide, but I'm not really understanding. So, here's the interested part.

---

I've a few questions.

1. Are the projected points $q_1$ and $q_2$ the points with respectively the screen coordinates $(x_1, y_1)$ and $(x_2, y_2)$, i.e., $q_1 = \begin{pmatrix} x_1 \\ y_1\end{pmatrix}$ and $q_2 = \begin{pmatrix} x_2 \\ y_2\end{pmatrix}$?

2. Regarding how to obtain the projected points. What I understand from the slide is that the projected $3D$ points are obtained by dividing all coordinates of $p_1$ (e.g.) by its $z$ coordinate, i.e., $p_1^z$, so that we obtain $q_1 = \begin{pmatrix} \frac{p_1^x}{p_1^z} \\ \frac{p_1^y}{p_1^z} \\ \frac{p_1^z}{p_1^z} \end{pmatrix} = \begin{pmatrix} \frac{p_1^x}{p_1^z} \\ \frac{p_1^y}{p_1^z} \\ 1 \end{pmatrix}$. So, I guess, that at this point, we obtain the sccreen coordinates by ignoring the $1$ as the 3rd coordinate. Is that right?

Answer 1

It's not 100% clear what the author means here, but I'll choose to interpret "screen coordinates" as "pixel coordinates". These would be related to the projected points by a 2D coordinate transformation.

You're correct that projection is done by dividing each component of a point by its $z$ component. (That's not quite true—actually, we usually use homogeneous coordinates, which have four components $xyzw$, and divide by the $w$ component, which has previously been set to eye-space $z$ by the projection matrix. This frees up the $z$ component of the vectors to be used for the z-buffer.) The projected points $q_i$ are then in "normalized device coordinates" or NDC space, which has a range of [−1, 1] on each axis.

To calculate the resulting pixel coordinates on the screen, we then need to perform a 2D scale-and-translate operation, to map the [−1, 1] range to the desired range of pixels. For example, if we want a 640×480 viewport, we could calculate $$x_i = 640 \cdot \frac{q^x_i + 1}{2} \\ y_i = 480 \cdot \frac{q^y_i + 1}{2}$$ You might also need to flip the $y$ axis in this transformation, for instance if the NDC space is Y-up but the pixel coordinates are Y-down.