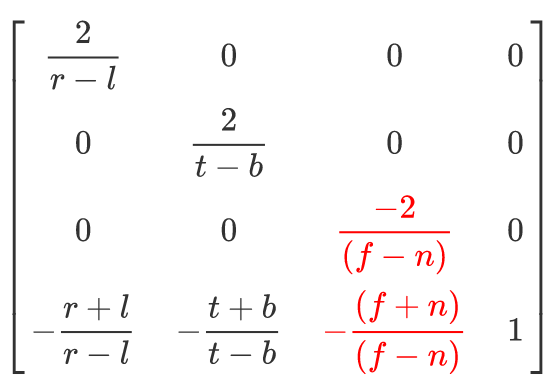The projection matrix tells us how to draw an image. But how does it tell us this? Well it tells us what the original vectors are like when drawn on screen. The axonometric projections have fixed direction vectors, isometric is just one of them.
Now there is a entirely visually verifiable correlation between the matrix and the axes of your axonometric projection. You can offcourse also do this with rotations as others have suggested but its a very nonstraightforward way to achieve something you should be able to do by drawing the axes on a 2d surface.
Now lets take the standard 120 forward facing isometric axes with z up (offcourse any coordinate could be flitted and you could have Y up as well just change sign or swap rows in matrix. Covnention random happens to be this since I was doing architectural stuff last and architects like z up since they draw in the X-Y plane. Had i worked with machanical engineers it would be Y up):
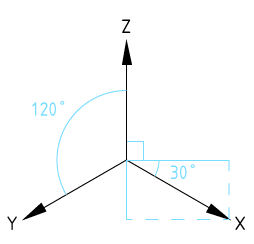
Each basis vector is just a row in the matrix. From this follows that in your camera matrix is of form:
$
\left[ {\begin{array}{cc}
cos(30°) & -sin(30°) & z_x & 0 \\
-cos(30°) & -sin(30°) & z_y & 0 \\
0 & 1 & z_z & 0 \\
c_x & c_y & c_z & 1 \\
\end{array} } \right] =
\left[ {\begin{array}{cc}
\sqrt{3}/2 & -1/2 & z_x & 0 \\
-\sqrt{3}/2 & -1/2 & z_y & 0 \\
0 & 1 & z_z & 0 \\
c_x & c_y & c_z & 1 \\
\end{array} } \right]
$
So the last column is 0 because it does not participate in a perspective. The variables $c$ represent the offset for your center. Then you may also want to multiply all the x y z components with a multiplier to fit your screen drawing coordinates, but that depends on your imaging system.
What about $z$? Well you want z values to be affected by your ground plane distance somehow. Now you could decompose all this to rotations to get a natural z value. Or you can just put in a value in $z_x$ and $z_y$ and see what happens its probably not all that important as long as your geometry does not clip by near and far planes.
Similarly since the vectors are just what you see you can make any axonometric projection by drawing your axes and feeding their 2d coordinates into your matrix.
NOTE: The matrix may be transposed depending on how your system calculation order works.