[Disclaimer: I think the following should work but have not actually coded it myself]
I couldn't think of a "trivial" method of producing a yes/no answer but the following would be a reasonable approach to a practical solution to the question.
Let's assume our curves are A(s) and B(t) with control points {A0, A1..An} and {B0,..Bm} respectively.
It seems to me that, given a pair of 2D Beziers for which we wish to determine do or don't intersect, there are six cases to consider:
Case where we can "trivially" determine they do not intersect.
Case where they intersect a finite number of times and we can "easily" determine they definitely intersect at least once (but we don't actually care where those intersections occur)
One of the Beziers is degenerate, i.e. a point (which will occur if all the control points are identical). We can assume we've already handled the case where both are points.
One or more of the curves are closed, e.g.. A0==An. To make life simpler, we'll subdivide such curves and start again.
There are an infinite number of points of intersection because each is subset of a "parent" Bezier and they overlap.
We aren't certain about the above cases and need further investigation
For the moment we'll ignore 3 and 4, but come back to them later.
Case 1
As you hint in your question, if the respective bounding boxes of the control points of A and B), don't intersect, then the curves can't intersect. Obviously this is a quick reject test but it's overly conservative.
As you probably know, with a Bezier curve, the convex hull of its control points forms a (tighter) bound on the curve. We can thus use the separating axis technique to decide if the hulls of A and B don't intersect. (e.g. as shown in Wikipedia:)

Case 2
If the case 1 test fails, you could then check for the "trivial" existence of an intersection. Now there are probably better ways to do this, but the following, relatively cheap, approach occurred to me:
Consider just curve A:

We know the curve starts at $A_0$, terminates at $A_n$, and will lie inside the convex hull. For simplicity let us compute the direction of the line segment $\overline{A_0A_n} $ and the compute the bounds on either side (i.e. take dot products of the remaining control points against the perpendicular to $\overline{A_0A_n}$).
If we do the same with curve B we get the following (possible) case:
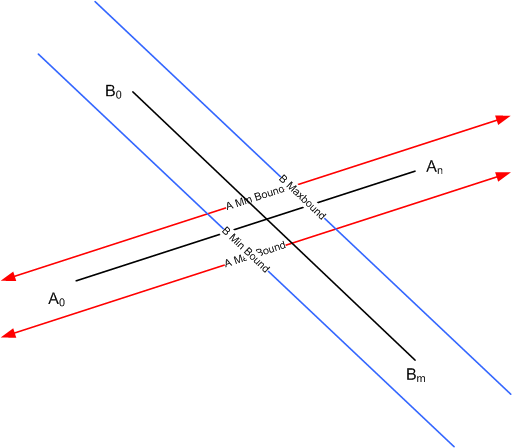
If we find $A_0$ and $A_n$ are outside opposite bounds of B and that $B_0$ and $B_m$ are on the outsides of the bounds of A, then, by the continuity of Beziers, there must be at least one intersection.
Case 6
If we can't immediately show either of the above cases, then split each of the Beziers into two "halves", i.e. $A^1, A^2, B^1, B^2$. This is relatively straightforward (left as an exercise to the reader) but is particularly trivial for quadratic Beziers:
Recursively compare the 4 combinations: $(A^1,B^1), (A^2, B^1)...(A^2, B^2)$.
Clearly if all pass case 1, there is no intersection.
If any fail 1, then continue with the rest of the tests with that reduced subset.
Case 3 & 5
This is where it becomes slightly more tedious.
If "case 3" gets past the "case 1" test, it seems to me that you need to solve for an actual intersection. Given that there is a simple process to map the N control points of a Bezier, A(s), to the N-1 points of the Bezier, A'(s), representing its 1st derivative then (provided care is taken for the relatively rare, so-called "degenerate" situations where the 1st derivative does to zero), then Newton iteration (on one dimension) could be used to find potential solutions.
Note also that, since the control points of A'(s) are a bound on the derivative values, there is the potential to do early elimination of some cases.
Case 5 seems relatively unlikely, so perhaps only if after a few recursions there is no conclusive proof, one could try each end point of A against curve B and vice versa. This would only give a proof of intersection - not a proof of non-intersection.


