6
$\begingroup$

I need to draw a logarithmic spiral (or close approximation) whose vertices are equally spaced, such that the lines between any two consecutive vertices are of equal length.

(Actually, that spiral is just the basis for something a little more complex, but equally spaced incrementation is what matters right now.)

The basis for the spiral is $$r=e^{0.25 \theta}$$ where $r$ is the radius and $\theta$ is the angle from the $x$ axis.

So it's easy to step around the spiral by a given angle, but obviously the actual length of any line between two vertices (defined by change in angle $\Delta\theta$) will vary with the radius.

How can I step along the arc of the spiral by a set length (say $0.1$ arbitrary units) and get the right coordinates (polar or Cartesian)?

Improve this question
$\endgroup$
6
  • $\begingroup$ Does this need to be fast or can it be done offline in advance? $\endgroup$
    – Alan Wolfe
    Commented Oct 16, 2017 at 19:59
  • $\begingroup$ It doesn't need to be especially fast. $\endgroup$
    – Solar Granulation
    Commented Oct 16, 2017 at 20:00
  • $\begingroup$ Do the segments need to be exactly equal length (within limitations of floating point etc) or can they be approximately equal? Like equal within some epsilon? $\endgroup$
    – Alan Wolfe
    Commented Oct 16, 2017 at 20:02
  • $\begingroup$ Interesting point. Some approximation is probably okay, but I'd prefer to maximise precision. $\endgroup$
    – Solar Granulation
    Commented Oct 16, 2017 at 20:04
  • $\begingroup$ Do you want equal length segments or segments that represent equal arc lengths? $\endgroup$
    – Sebastián Mestre
    Commented Oct 17, 2017 at 0:44

1 Answer 1

Reset to default
9
$\begingroup$

Since a logarithmic spiral is defined by

$r=e^{a\cdot\theta}$,

the inverse of the equation is this:

$\theta=\frac{\ln{r}}{a}$.

If we want to be able to control our step value, we can multiply it by a scalar ($a\cdot k$) before taking the logarithm, like so:

$\theta=\frac{\ln (ak\cdot r)}{a}$

Therefore, if we take the natural log of theta multiplied by the scalar and $a$, then divide the whole thing by $a$ before plugging it in to the equation, we will get equally stepped vertices on the logarithmic spiral. Image:

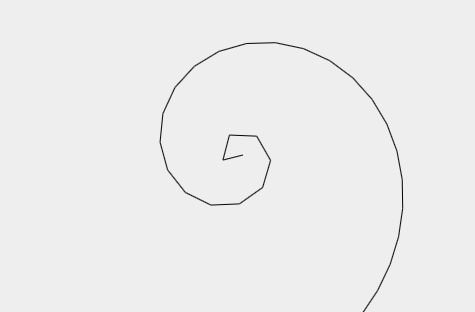
enter image description here

I generated this with some JavaScript code, which you can find in this JSFiddle.

You can also try it out interactively on Desmos.

This looks good initially, but let's analyze what's going on here a little deeper.

Here is a list of the distance between each consecutive pair of points, starting at the origin and spiraling outward:

20.6834877876017
25.8265879847751
27.3020264943519
27.9155365639631
28.2274404549991
28.4072329605331
28.5201842104742
28.5957343926866
28.6487385499231
28.6873487186944
28.7163404861061
28.7386617798632
28.7562121095165
28.7702600892245
28.7816791255158
28.7910864406300
28.7989282185451
28.8055334775293
28.8111491387549
28.8159634339811
28.8201218817722
28.8237384111142
28.8269032465565
28.8296885891879
28.8321527704665
28.8343433306399
28.8362993285731
28.8380530946981
28.8396315754034
28.8410573741728
28.8423495651939
28.8435243345428
28.8445954894748
28.8455748659368
28.8464726569062
28.8472976786488
28.8480575879711
28.8487590604974
28.8494079377702
28.8500093492502
28.8505678139929
28.8510873257846
28.8515714247378
28.8520232577561
28.8524456298024
28.8528410475339
28.8532117565801
28.8535597734869
28.8538869132081
28.8541948128041
28.8544849519687
28.8547586708355
28.8550171854833
28.8552616014621
28.8554929256347
28.8557120765606
28.8559198936319
28.8561171451194
28.8563045352784
28.8564827106597
28.8566522656712
28.8568137475608
28.8569676608282
28.8571144711722
28.8572546090145
28.8573884726640
28.8575164311457
28.8576388267439
28.8577559773038
28.8578681782850
28.8579757046362
28.8580788124715
28.8581777406078
28.8582727119331
28.8583639346897
28.8584516035825
28.8585359008445
28.8586169971792
28.8586950526148
28.8587702173116
28.8588426322609
28.8589124299770
28.8589797350751
28.8590446648521
28.8591073297703
28.8591678339633
28.8592262756316
28.8592827474659
28.8593373370150
28.8593901270040
28.8594411956751
28.8594906170532
28.8595384612331
28.8595847946223
28.8596296801627
28.8596731775581
28.8597153434631
28.8597562316691
28.8597958932898
28.8598343768895
28.8598717286685
28.8599079925824
28.8599432104601
28.8599774221564
28.8600106656362
28.8600429770912
28.8600743910437
28.8601049404189
28.8601346566671
28.8601635697958
28.8601917084984
28.8602191001836
28.8602457710551
28.8602717461905
28.8602970495771
28.8603217041829
28.8603457319945
28.8603691540817
28.8603919906349
28.8604142610140
28.8604359837728
28.8604571767236
28.8604778569632
28.8604980408853
28.8605177442508
28.860536982199 
28.8605557692684
28.8605741194424
28.8605920461606
28.8606095623542
28.8606266804642
28.8606434124606
28.8606597698673
28.8606757637894
28.8606914049092
28.8607067035307
28.8607216695845
28.8607363126319
28.8607506419126
28.8607646663229
28.8607783944441
28.8607918345695
28.8608049947040
28.8608178825589
28.8608305056052
28.8608428710407
28.8608549858324
28.8608668567086
28.8608784901671
28.8608898925065
28.8609010698021
28.8609120279379
28.8609227726126
28.8609333093227
28.8609436434116
28.8609537800355
28.8609637242006
28.8609734807368
28.8609830543426
28.8609924495660
28.8610016707985
28.8610107223272
28.8610196082769
28.8610283326623
28.8610368993920
28.8610453122201
28.8610535748236
28.8610616907611
28.8610696634759
28.8610774963191
28.8610851925415
28.8610927553168
28.8611001876897
28.8611074926601
28.8611146731067
28.8611217318631
28.8611286716494
28.8611354951266
28.8611422048820
28.8611488034280
28.8611552932030
28.8611616766001
28.8611679559112
28.8611741333984
28.8611802112609
28.8611861916049
28.8611920765250
28.8611978680366
28.8612035681006
28.8612091786388
28.8612147015119
28.8612201385397
28.8612254914896
28.8612307620868
28.8612359520116
28.8612410629093
28.8612460963629
28.8612510539384
28.8612559371502

The first data point is an outlier caused by the spiral almost "lapping" itself. As the spiral progresses, we can see the the distances get closer and closer to the same distance.

This is because we are sampling the curve in increments that cause equal lengths along the curve, not equal lengths between consecutive points. As the spiral gets bigger and bigger, the curve will get shallower and shallower, so the distances will get closer and closer to each other.

Improve this answer
$\endgroup$
3
  • $\begingroup$ That being said, this approach does mean that the value of a influences both line length and turn rate. I really need those to be separate terms. $\endgroup$
    – Solar Granulation
    Commented Oct 17, 2017 at 11:01
  • $\begingroup$ @SolarGranulation I just added that, and updated the JSFiddle and Desmos examples to reflect that. $\endgroup$
    – Scott Milner
    Commented Oct 18, 2017 at 0:10
  • $\begingroup$ "The logarithmic spiral is a spiral whose polar equation is given by $r=ae^{b \theta}$, where r is the distance from the origin, $\theta$ is the angle from the X axis" (Wolfram math). that's what $r$ is, which you're incrementing by constant steps. it's not the distance along the curve. $\endgroup$
    – Will Ness
    Commented Aug 21, 2023 at 16:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.